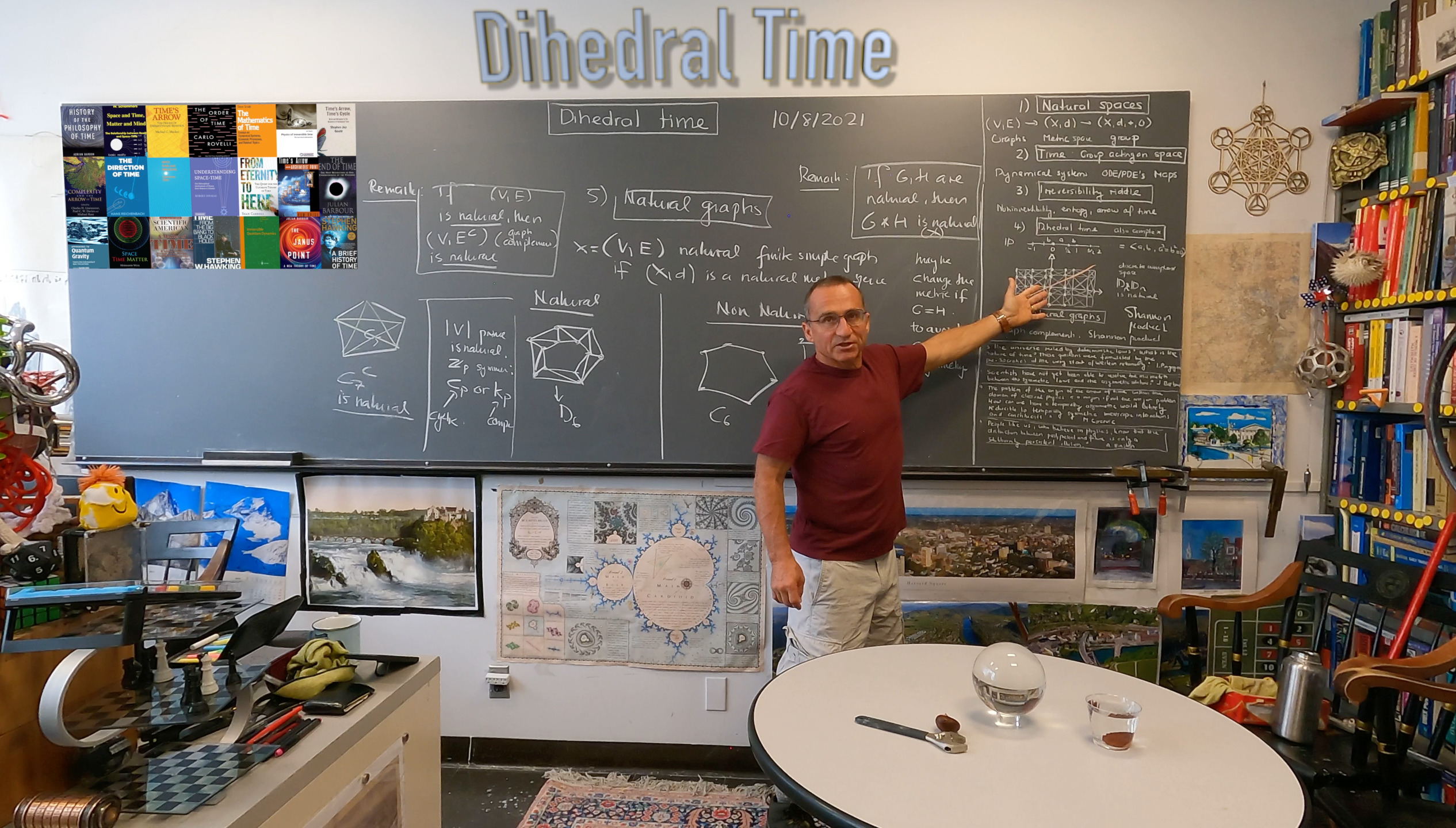
The dihedral group is a natural discrete time line. One reason is purely mathematical: unlike the integers, the group is determined by a metric space. An other reason is that it is generated by involutions, structures which are at the heart of any geometry or quantum field theory. One can also see it as the space of half integers. The numbers are …,bab,ba,b,0,a,ab,aba,… The even parts are the integers ab=1,abab=2,ababab=3, ba=-1,baba=-2,bababa=-3 etc. The odd parts are the half integers a=1/2,aba=3/2, b=-1/2,bab=-3/2. The half integers obviously are reflections while the integers are translations. One can be led to the half integers also when looking for a discrete calculus in which the Taylor theorem works symmetrically. Instead of taking the derivative f(x+1)-f(x), one can take the derivative f(x+1/2)-f(x-1/2). When evaluated on integers, it taps into half integers, when evaluated on half integers it taps into integers. We will come back to the calculus.