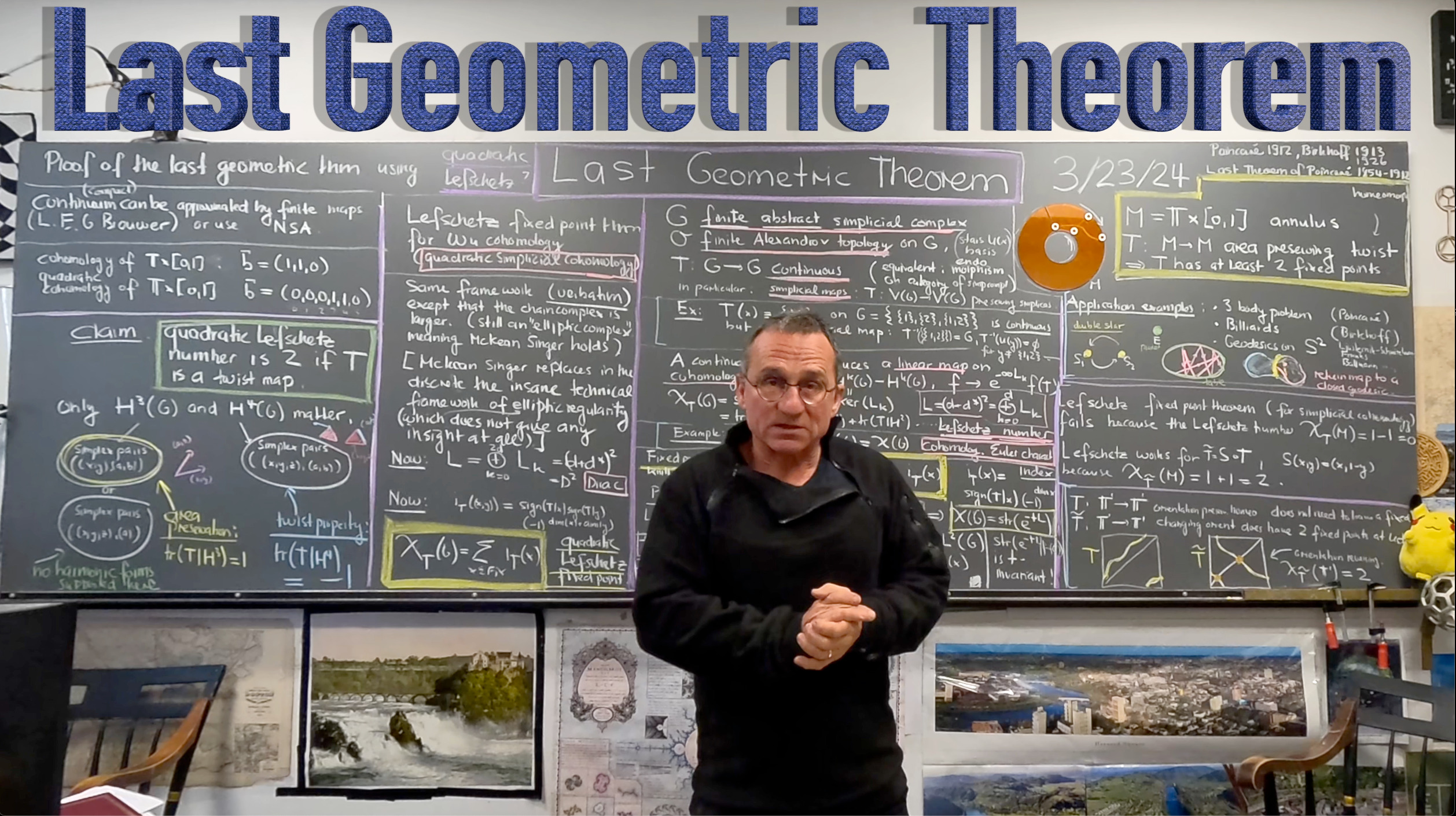
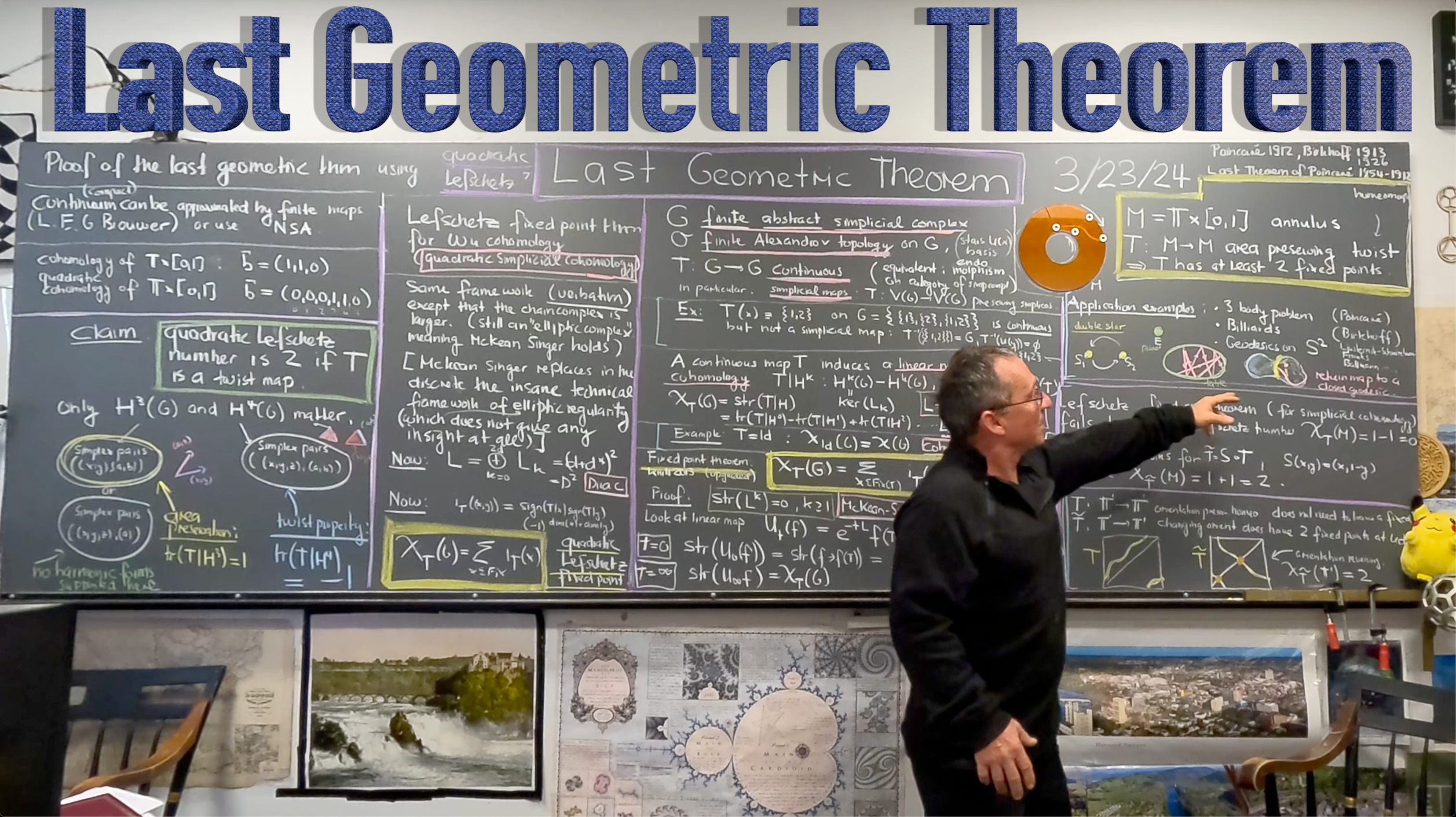
The last geometric theorem of Poincare was conceived shortly before the death of Poincare. Poincare had a prostate problem when he was 58 and went to surgery in 1912 which he did not survive. Fortunately his last theorem was sent to an Italian journal two weeks before he died, but Poincare did not prove it yet even so he had a good idea. There was a paper of 2021 of Hubbard with a student pursuing Poincare’s original path. Also Gole and Hall have shown such a proof. Birkhoff did not quite succeed yet also in 1913 to prove the theorem completely, especially establishing the second fixed point. He finished this in 1926 (there are some who still think that also this paper was not quite tight). I myself learned the theorem from a paper of Neumann (a son of two famous algebraists (husband and wife) working in geometric group theory) and Brown (who would win the Veblen prize together with Mazur in the context of the Schoenfliess problem. A small world!). Actually, I had to present the proof in a Moser seminar in 1986. It had been the third analysis seminar and I had learned that semester that for graduation, I needed an other pro-seminar, one in algebra. So, I had to present a theorem in Lie algebras (Engel’s theorem) in a pro seminar of Mislin and bail out of the Moser seminar which supposedly made Moser a bit upset. The theorem is tough to prove in all details in one hour and I would have needed an other hour to do it properly.
In this presentation, I wonder whether the last geometric theorem could be proven from the Lefschetz theorem for quadratic cohomology. It would be very elegant as the Lefschetz theorem itself is very elegant. For spaces with trivial simplicial cohomology like contractible spaces, there is always a fixed point for example. This is the Brouwer fixed point theorem.