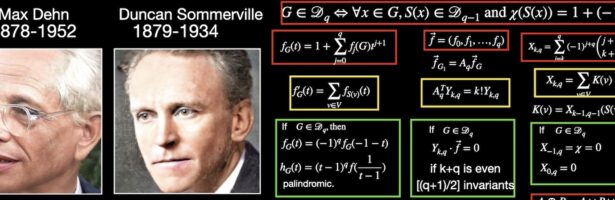
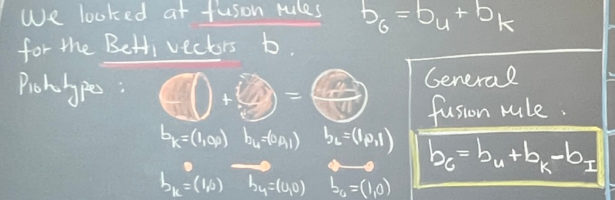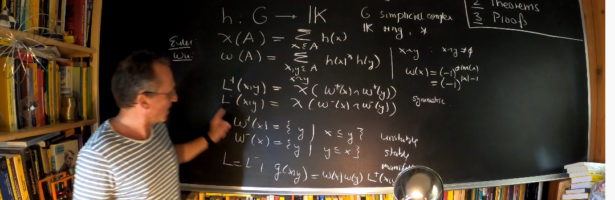Elements of Finite Geometry
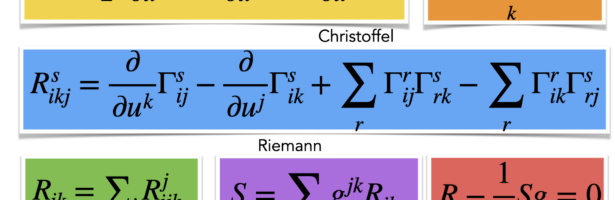
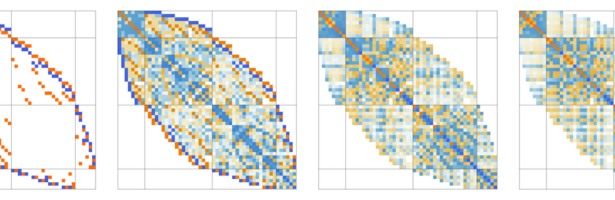
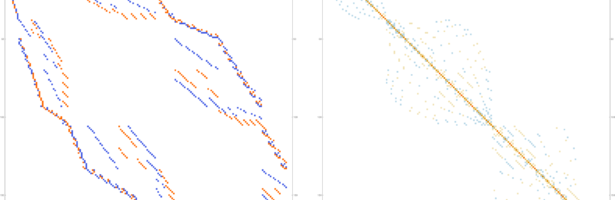
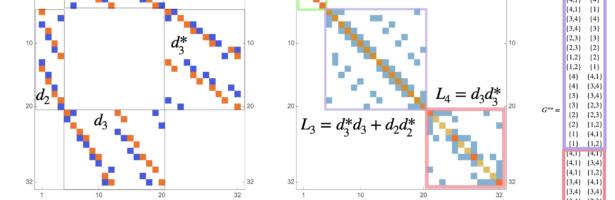
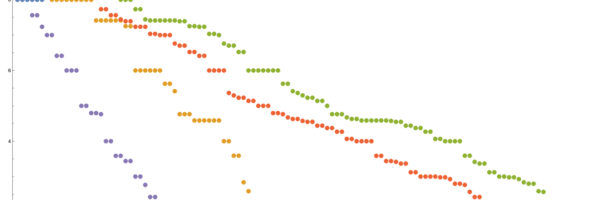
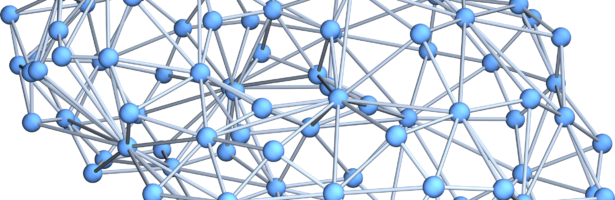
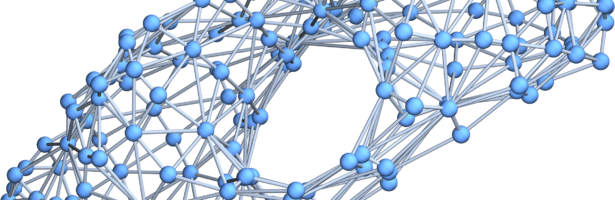
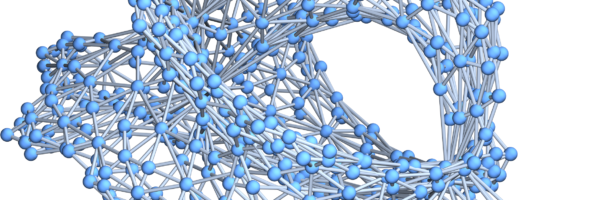
While thinking about some fundamental parts in the story of Dehn-Sommerville, I decided to make a bit of an exercise in imagining how Euclid would have done finite geometry. The result is given in the movie below. Euclid’s elements is undoubtedly one of the milestones in the whole of mathematics …