A central limit theorem for high risk

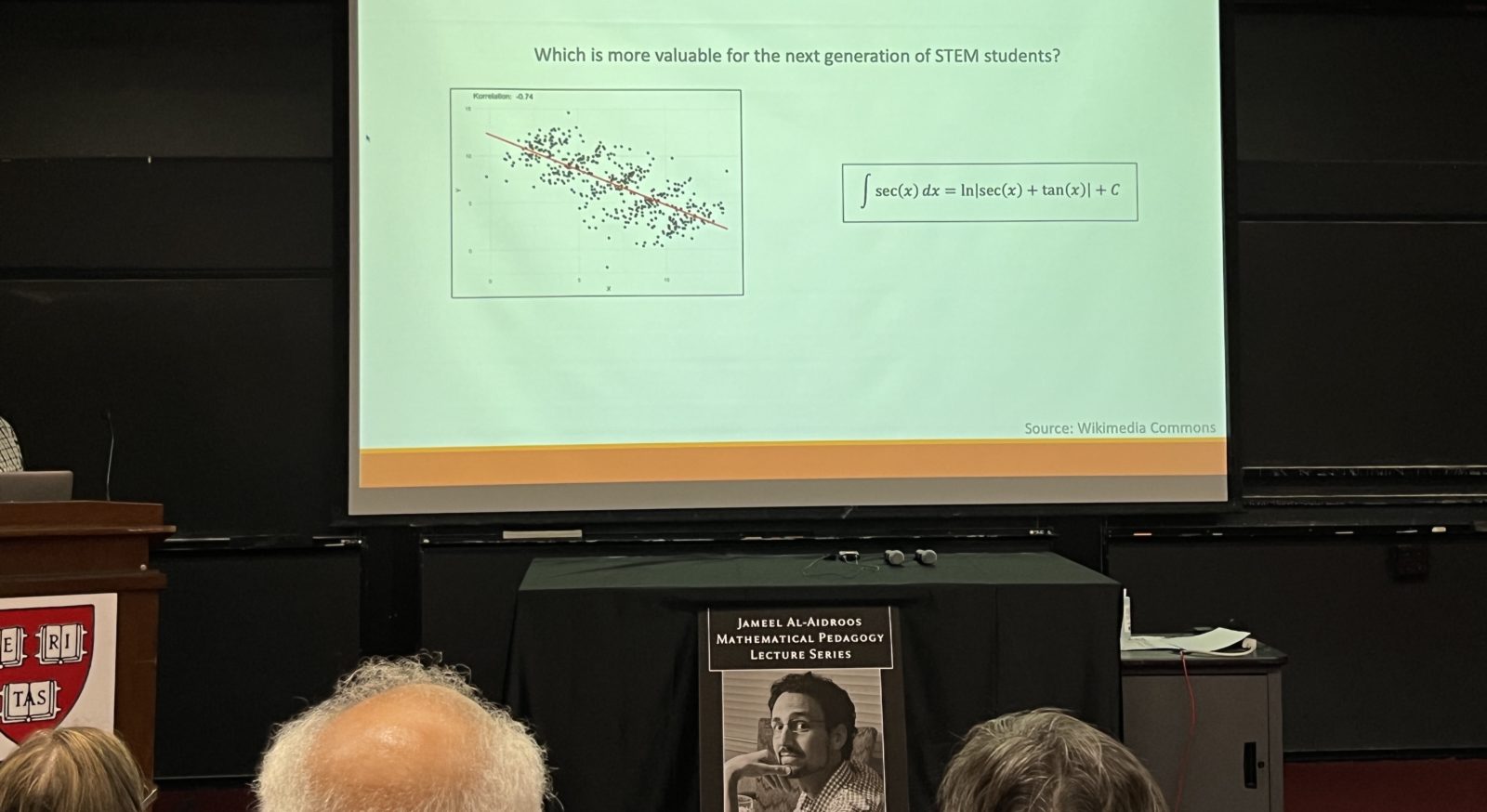
There were two triggers for this: first of all the KAM fixed point equation is for c=0 a renormalization map which matters in probability theory, in particular for the Cauchy distribution which is a fixed point under the map which gets from a distribution the distribution of [X+Y]/2, where X,Y have the distribution. Proving that the fixed point is an attractor for some basin of attraction is a central limit theorem. It is something, I had encountered when looking at almost periodic Cauchy distributed random processes, something which has many relations with other mathematical topics, like modular forms, the theory of partitions in number theory, etc etc. Then there was a talk last Monday at the Memorial conference for Jameel in which Aubrey Clayton gave talk about http://cup.columbia.edu/book/bernoullis-fallacy/9780231199940 the Bernoulli Fallacy. It was a great talk except that the value of statistics was laughing a bit about calculus. I objected after the talk to Abrey in person. While I find his book extremely important and his talk fantastic, the education about basic statistical fallacies is important. But basic mathematics like logica or calculus is even more important. Without hard mathematics, the fight will be hopeless. From reading in his book, it becomes clear that most of the confusion in these matters is actually abuse of basic logic. For example, (one can see this on the left hand side of the slide), it is possible that in every category of a class of objects a specific averaged quantity goes down but that overall, the average goes up simply because the weights are shifting towards the higher average. You can see this in calculus too. If you have an object with density it is possible to deform the situation so that in every slice the mass goes down even so the total mass goes up. The reason is that that the part where the high density is can become bigger and more relevant. This has little to do with knowing about statistics. It is just basic raw intelligence and knowing what accumulated and averaged quantities are. One learns this in calculus! And in general in any math course.

The central limit theorem is the fundamental theorem in probability theory. (I argued once https://people.math.harvard.edu/~knill/graphgeometry/papers/fundamental.pdf why not the law of large numbers). For a proof see the book “probability theory and stochastic processes” (PDF) https://people.math.harvard.edu/~knill/books/KnillProbability.pdf, The Cauchy version result was marginally mentioned in Birkhoff sums of cot function (2012) https://arxiv.org/abs/1206.5458, where also standard Cauchy distributed random variables are summed but where we have almost periodic correlations.See also a talk from 2014 at BU https://people.math.harvard.edu/~knill/slides/bu/boston.pdf