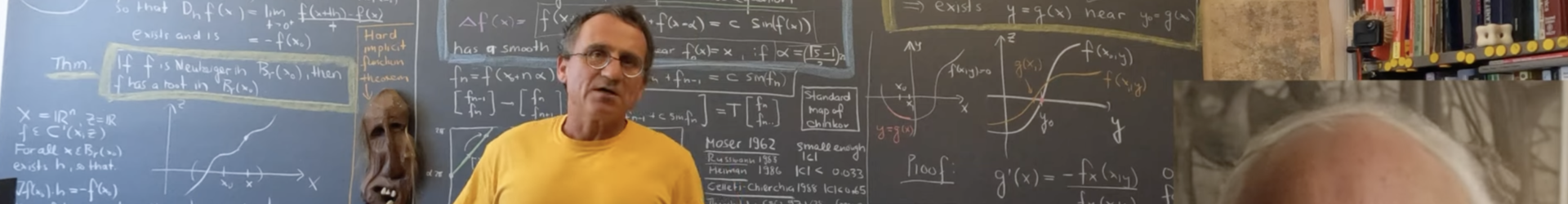A KAM Challenge - Quantum Calculus

Looking at images of the Standard map (see https://people.math.harvard.edu/~knill/technology/chirikov for a Javascript animation) is fascinating because the pictures illustrate lots of unsolved enigmas. The picture shows stability and instability mixed. Invariant curves are obtained by solving the functional equation $f(x+\alpha) - 2f(x) + f(x-\alpha) = c \sin(f(x))$ for smooth functions. KAM theory establishes that. In the movie, I illustrate a bit, how we were trying to look for a simpler proof of this relation. One can see the functional equation also as a fixed point problem for the operator $T(f)(x) = [f(x+\alpha) + f(x-\alpha) - c \sin(f(x))]/2$ . This is of course not a contraction so that a Banach fixed point theorem does not work. We can also not use an other “easy theorem” like a Lefschetz formula to find fixed points near $f(x)=x$ which is a solution at $c=0$ . The fixed point equation $Tf(x) = [f(x+\alpha) + f(x-\alpha)]/2$ by the way leads to the next video on October 22 about a central limit theorem. When looking at a random variable and averaging two IID copies of it, one gets a new distribution. The Cauchy distribution is a fixed point of this renormalization map. One calls this a stable distribution.

Back to this video: it is organized around the implicit function theorem, one of the most important theorems in mathematics. The video also relates back to the last video about intersection and incidence calculus relations and I will come back to this. It is extremely interesting that when changing the Laplacian to an intersection Laplacian that we do not need any hard implicit function theorem.

Author: oliverknill