As Hardy once pointed out in his apology, “languages die but mathematical ideas do not”. Hardy added that a mathematician “]has the best chance of becoming immortal whatever the word immortal might mean”. Let me illustrate this with three mathematicians, all teachers of mine, who have earned more than immortality. Specker is the S from Kochen-Specker, Moser is the M from Kolmogorov-Arnold-Moser and Lanford is the L from Dobrushin-Lanford-Rulle. Being entombed in an acronym is probably the closest one can get to become a God. But the main reason to include this here in a blog on quantum calculus is that all three had strong interests in the connections between the discrete and the continuum. Maybe for different reasons: Specker was more fundamentally interested in the foundations of mathematics and discrete approaches like Kustannheimos, Lanford was interested in what it means when we simulate physics and mathematics with computers which are finite entities, Moser through his background with Siegel was a rather classical and conservative mathematician with strong interests also in number theory and algebraic geometry like simulations of twist maps on finite fields as the astronomer Rannou did first.
Ernst Specker
Ernst Specker is probably best known through the Kochen-Specker theorem. It is one of the few theorems which also non-mathematicians are fascinated with and which I had been asked about personally by people about who are not mathematicians. The reason for the fascination with that topic that it deals with the fundamental question of what quantum mechanics. It taps into the meaning of reality, the meaning of what it means to think using logic, the meaning of our existence. To back up the claim that this is of interest to humanity, one can observe that there are dozens of books about quantum reality, Schroedinger paradoxa, many world interpretations, the meaning of time and space etc. and that talks about the subject are usually packed (Froehlich at Harvard math department was one of the few talks I have seen there where Hall 509 was unable to hold all of the audience, similarly to talks of Nima Arkani-Hamed or Lee Smolin or Brian Green). Specker is also a connection to Heinz Hopf, as Specker was initially a topologist as a student of Hopf. Specker is a legend also because he was politically outspoken and had strong opinions about what it means to be free human being. Like Grothendieck, he was an independent thinker in any issues, also politically, even politically active on rallies, exposing Gestapo type police activities in the classroom (yes, also in Switzerland, there was a time, when political police would visit classrooms to expose “subversive teaching”. Specker had a good method to get rid of this scum: he would ask them mathematiclal questions). No country, neither the US nor Switzerland are immune against the dangers of fashism (a development which even started in the US recently, even with liberal seemingly reasonable people starting to flirt and admire autocratic structures in order to control of the population). Fortunately, both countries, due to similar strong historical ties to what it means to be free and independent have been able to avoid such a fate so far (Switzerland with considerable luck). Other countries have not been so lucky. An other legend tells that when Raoul Bott (an other legendary and immortal mathematician) was producing conjectures in topology almost every day, Specker would be the sparring partner, finding counter examples. Specker is a connection to the more playful part of mathematics, to riddles and games and the foundations as well as to linear algebra (as I had a two semester linear algebra course from Specker). Finally, quite a few grand children of Hopf were teachers of mine, including Roland Staerk (a student of Benno Eckman who was a student of Hopf), my high school teacher who was vastly responsible why I got interested in mathematics at all. Specker was very interested in the foundations of mathematics and especially the interplay between continuum and discrete, between finite and infinite. The Kochen-Specker theorem is a discrete, very concrete illustration of what quantum mechanical measurements mean. Besides that, Specker also mentioned in his classes work of Paul Edwin Kustaanheimo who had been working on though experiments in which one uses finite fields rather than the real or complex numbers.

Oscar Lanford
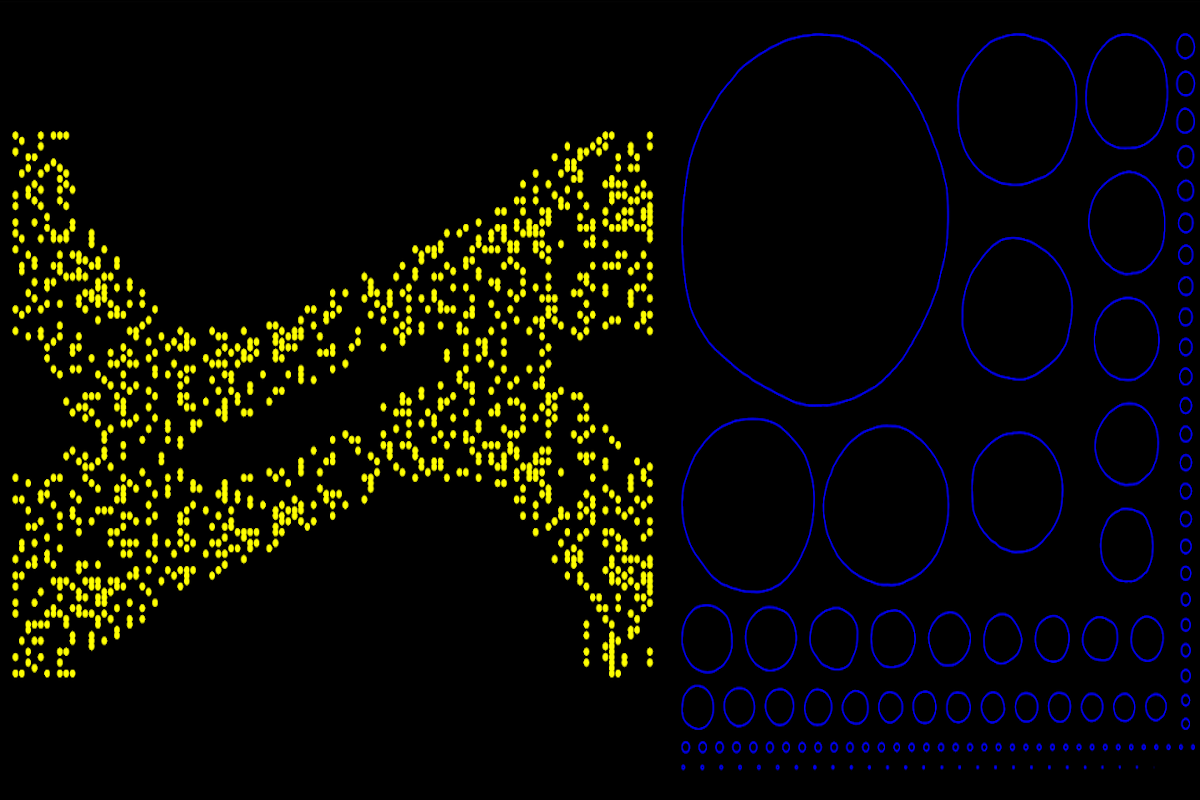
Oscar Lanford is the L of DLR, the Dobrushin Lanford Ruelle equations. He was also the first to prove the Feigenbaum conjecture (with a remarkable computer assisted proof). Lanford was my PhD Dad and a link to an other extremely fascinating community. Lanford’s PhD dad was Arthur Wightman who had other students important to me like postdoc mentors Barry Simon or Rafael de la Llave. The “father of Wightman” John Wheeler is immortal not only through notions like Black holes but also because Wheeler had many important students, like Hug Everett, Robert Geroch, Warner Miller, Charles Misner, Kip Thorne , Robert Wald or Richard Feynman. This is a community, which has strong ties to physics. While Oscar Lanford grew up as a quantum field theorist, he moved to pure mathematics and got later more and more interested also in discrete mathematics and the statistical mechanics related to number theory. Later work on discrete approximations of dynamical systems by periodic transformations is not so well known. The interest of Lanford was certainly also motivated by computer arithmetic, whichcomes in when doing computer assisted proofs like rigorous in analysis, where one replaces everything with rational numbers so that no rounding errors matter and the tedious estimates could still be done by hand if one had the time. It is important when working with rigorous mathematics with the computer that one only uses integer arithmetic so that one can use rational numbers as interval boundaries. In other words, instead of the field of complex numbers, one works in number fields. Naturally, this has ties to very classical topics in number theory. When working with computer simulations, one also gets naturally interested in the foundations of mathematics as one has to contemplate what it means to make computations, whether it is acceptable to make proofs in which silicon replaces brain cells, and how we value such proofs. What does it mean to prove a theorem with the help of a computer? Can such a proof be considered correct? A bit independent of this is the question what does it mean if we simulate an ordinary or partial differential equation with a computer. On a computer, any deterministic system is periodic. What happens if we add randomness? Is this justified as a numerical scheme? In that case, one gets close to an other mathematical field in which Lanford was working in: statistical mechanics. The Bolzman equations for example are a stochastic model approximating much more difficult partial differential equations. Stochastic systems are more accessible to mathematics as one can then use tools from probability theory. In some sense, probability theory smooths out singularities as exceptional cases have now probability zero. Such tools are always used by physicists but much of the physics literature which deals with stochastic assumptions, like mean field theories are not mathematically rigorous. To do rigorous mean field theories, means to do renormalization type approaches which in general invokes heavy analysis. Already establishing existence of solutions of stochastic multi-particle systems like the Bolzman equation requires heavy analytic tools. The transition of using a stochastic model to simulate a deterministic system is similar than using a finite model to simulate it.
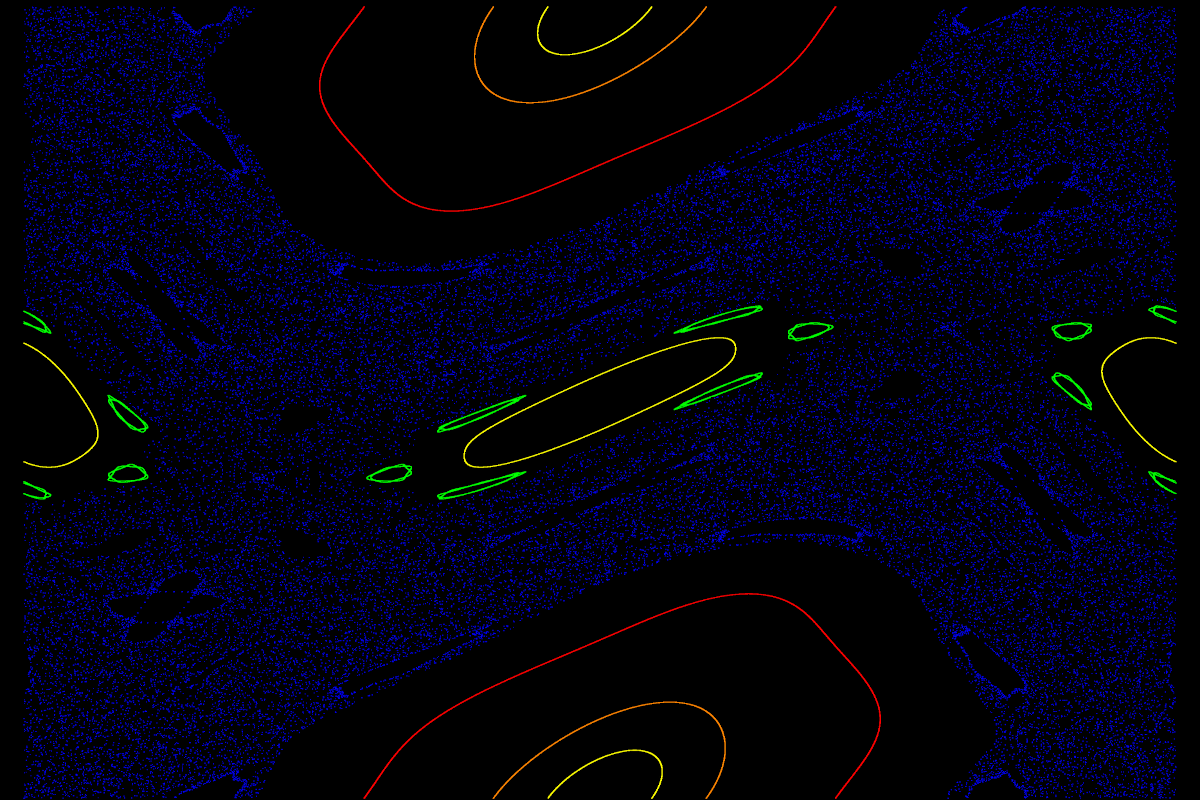
Juergen Moser
Juergen Moser is the M of KAM theory. Also the KAM theorem has reached fame far beyond the mathematics community. KAM is the reason why we exist, why our solar system appears like a clock despite the fact that it is a chaotic system (already a three body problem is no more integrable and very complicated in principle). KAM is also the reason why clocks move so regularly, even-so the underlying systems are driven oscillators which in general are non-integrable and very complicated. KAM is responsible for the stability of the van Allen belts which are crucial for the survival of the earth. (Without the shield of the magnetic field, our atmosphere would have been blown away by the solar wind similarly as it was on Mars. KAM gives order in the disorder. The particles in the van Allen belt have been measured to stay there trapped for months, even if the particle oscillates back and forth between the poles with speeds comparable with the speed of light. People like order and dislike chaos and that is maybe why KAM is so important. We like calm weather and dislike strong storms. Also, Moser was a world citizen, connected both to the West and theEast. Moser once was able to bring Vladimir Arnold to Zurich in 1993, when I had been a graduate student there. I had gone to that talk naturally. The title had been“plane curves, their invariants, perestoikas and classifications” and is still available. The book of Arnold on classical mechanics had been my bible since my sophomore year as it had been used as a textbook in a classical mechanics course of Juerg Froehlich. Froehlich’s course (he had at that time just recently come back from IHES) had been one of the most inspiring in my college years and sparked also more interest into physics. Moser is also a connection to Siegel, to Kolmogorov and to Arnold. Unfortunately, I never met Kolmogorov but I know some who have. Komogorov had a strong friendship to Pavel Alexandroff who also had a strong connection and personal ties to Heinz Hopf until very close to the death of Hopf (they also wrote fantastic books together). Not so well known is that Moser was also very interested in discrete number theoretical stuff. Of course, the obvious explanation is Siegel, who was a strong number theorist. Similarly as Gauss, who worked on astronomical problems and number theoretical problems, also Siegel had interest there. Moser was very much interested also in algebraic geometry, one reason of course the strong ties of integrable systems with algebraic geometry. (He has a fantastic lecture notes about integrable systems which I think are still the best today on the subject of integrable systems.) Whenever one has an integrable system one has either a scattering situation, or then an algebraic geometric background, where divisors move and are described by linear motions on a Jacobian variety via Abel transforms. I had tried to use such integrable Toda systems to prove a notorious open problem in ergodic theory which got booted up during my undergraduate thesis: the problem of positive entropy. In any case, very classical complex analysis (like the Abel-Jacobi transform) comes in in close contact to very concrete physics like Plasma physics, celestial mechanics or fluid dynamics. And these are all subjects where KAM matters.