What is space?
In Spring 2021, prompted by work on graph complements of circular graphs, I started to think more about discrete manifolds. One can see the definition on this video on discrete homotopy manifolds. In the spring of 2022, the definition got slightly modified and the name homotopy manifold changed to soft manifolds. Definitions should be simple, produce theorems and applicable in the sense that one can compute with them. As pointed out already last year, the question What is Space? is silly in the sense that we can never answer it for obvious reasons: assume we would understand the Planck scale as in the movie ant man, (the link to a movie clip is here), it would still not be excluded that on some other scale like a different mathematics would work. It is not excluded that on such a scale again classical mathematical notions would work and that the quantum world is just an intermediate anomaly. We already have no idea what happens on a scale of
. The best electron ptychographic reconstructions work allow to see to a scale of
(example). Ernest Rutherford already probed the interior of atom using the gold foil experiment exploring the
scale of the nucleus. Scattering experiments explored in the 1960ies the structure of quarks which are of the size
. There is quite a bit to go to the Planck length
. For a mathematician of course things do not end at this scale. We can zoom with a computer into the Mandelbrot set too. (Here is a zoom which was done to
). But even if for some strange reason we would start to understand the scale
of our physical space, what happens on a scale
? Even if we would see some self similarity, this could be a temporary effect which disappears on a scale
. It is silly to ask for an ultimate answer. What can we do? We can measure. We can probe space on various scales and see whether we can figure out its nature. It turns out that on any scale we so far were able to see, space always is a manifold: it is locally a three dimensional Euclidean space meaning that a small sphere is a 2-dimensional sphere. A 2-dimensional sphere is a locally Euclidean space which has the property that a small sphere in it is a 1-dimensional sphere which becomes a contractible disk after removing a point. A one dimensional sphere is a locally Euclidean space which becomes a contractible interval after removing a point. There are no indications that space is locally discrete or a complicated foam like network. One has suggested many mathematical models which could describe a quantum space time but if we insist what we see in physics, then there is no indication at all yet. If we insist on restricting the universe to parts we can do measurements, then we could model space as a manifold with boundary. The boundary is the place until we can see, it also can include spheres around a black hole singularity.
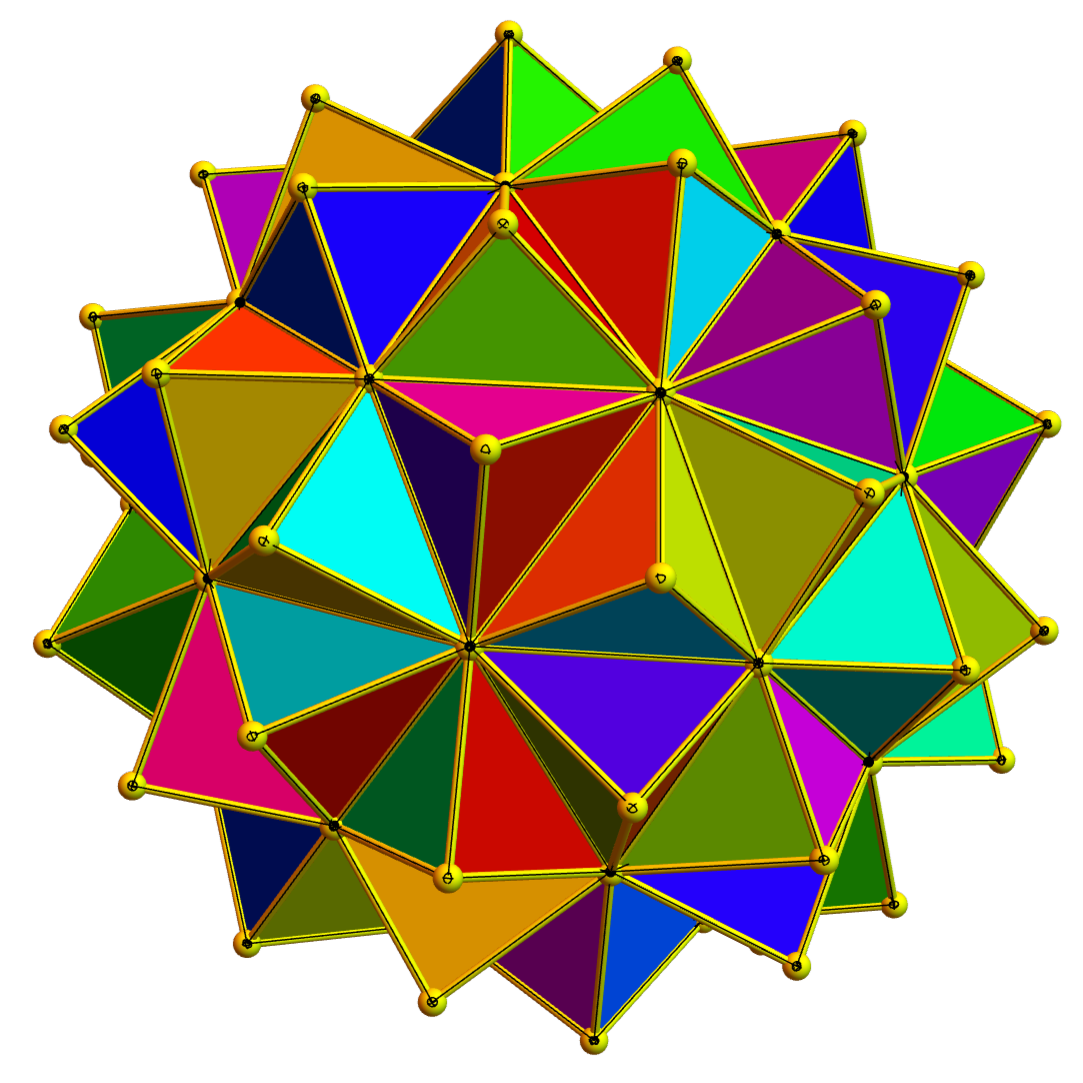
Echidnahedron 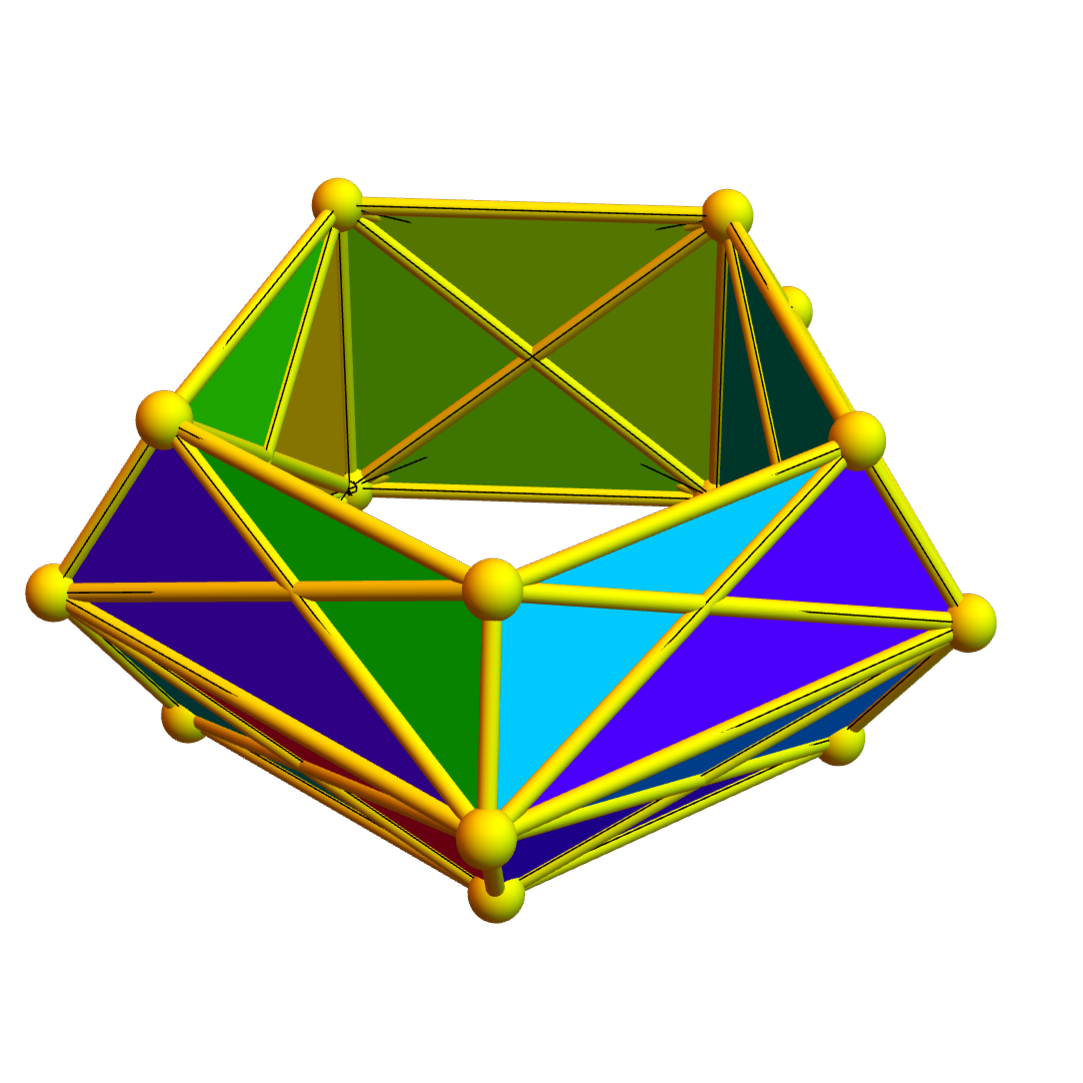
Shannon product of cyclic graph with complete graph 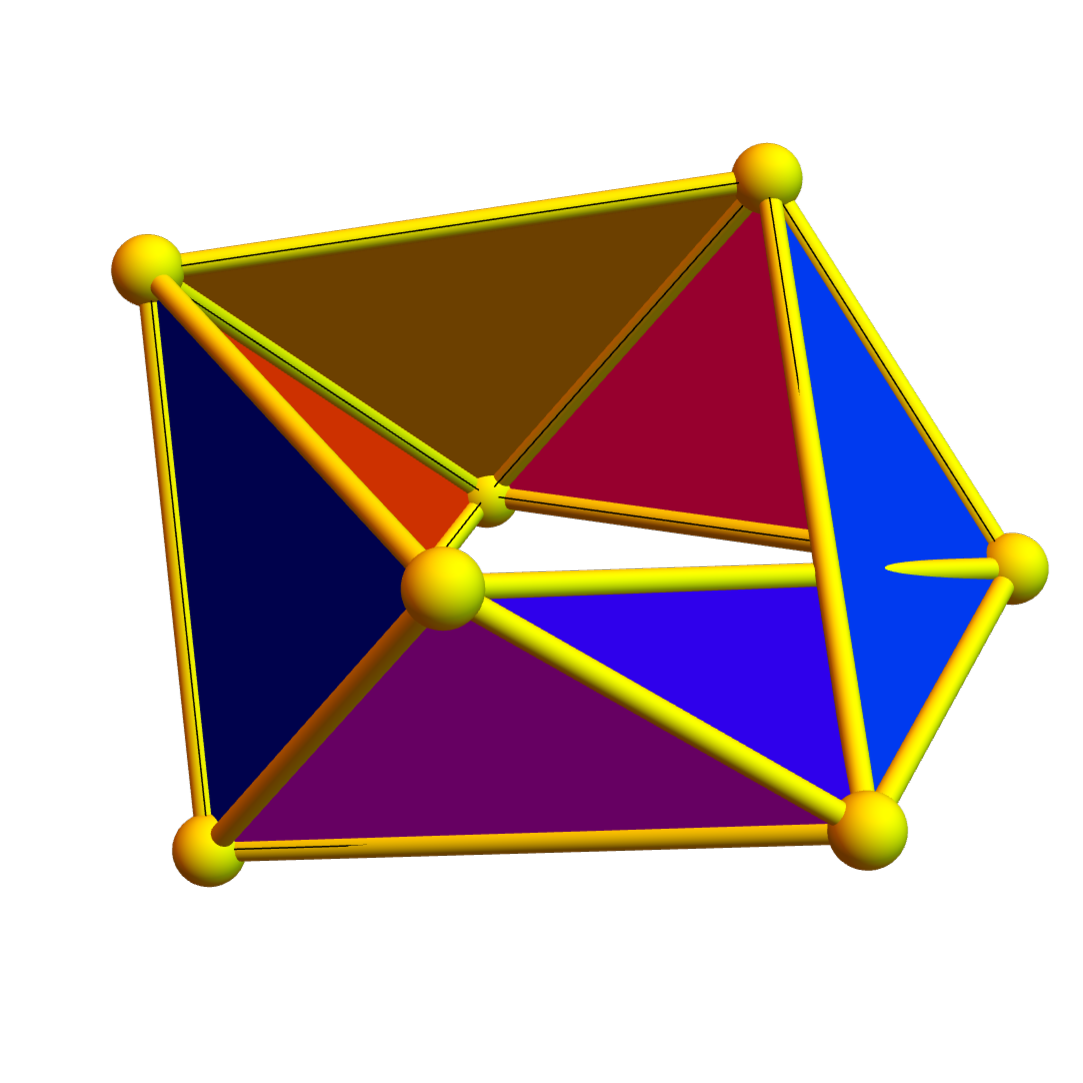
Graph complement of C7. 
Graph complement of c8 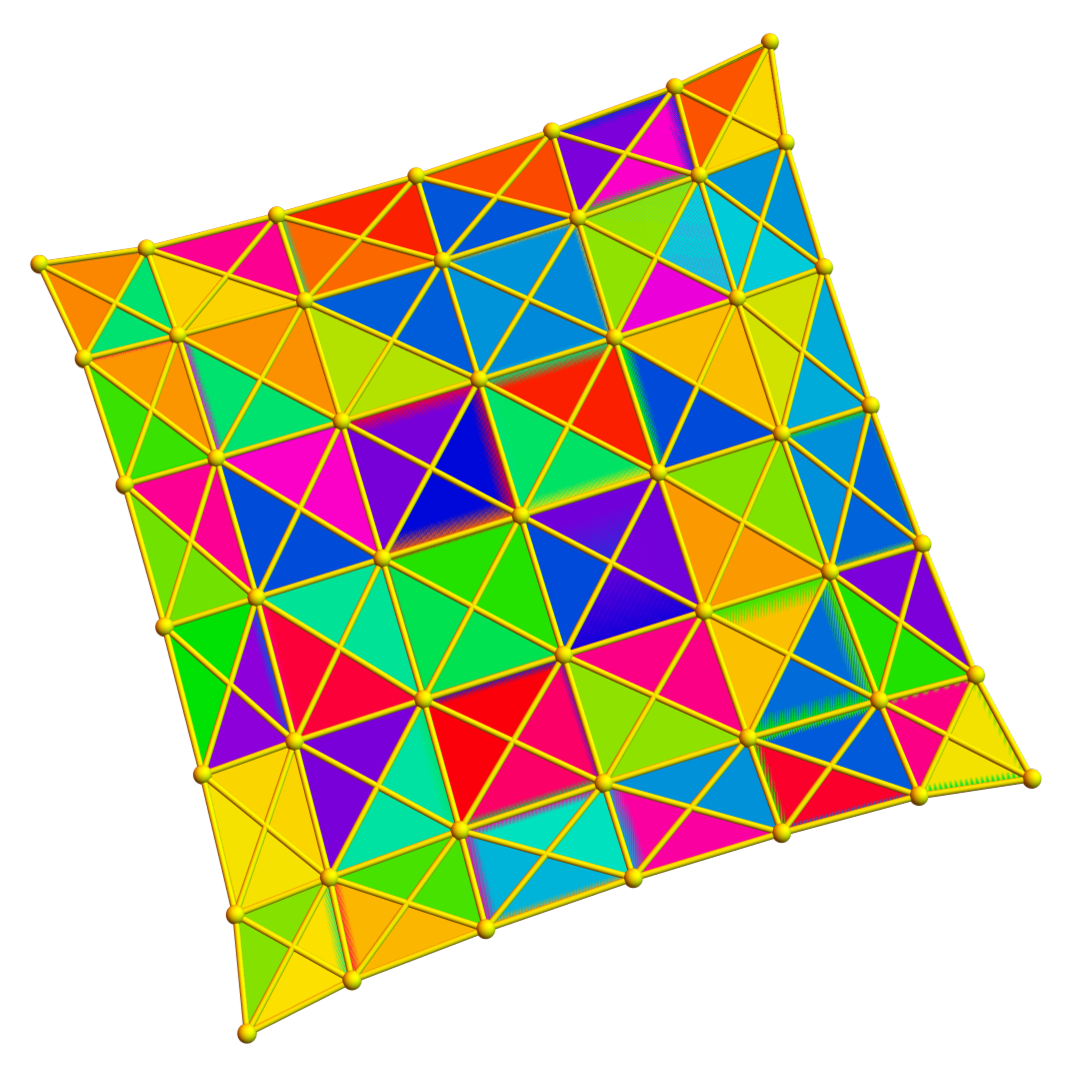
Shanno product of C6 * C6 
The connection graph of C4 is the unit sphere of the Shannon product of cylic graphs
Towards soft manifolds.
All we can do to explore space is to make distance measurements. We can determine as well as possible the position of as many points we possibly can do. What we then have is a finite set of points in space. This is what we do also when we measure out a surface like a face of a human. We can make measurements and produce a point cloud which models a two dimensional surface. If we do that we don’t get a nice crystal type polyhedron, we get a messy point cloud which is then simplified using mesh algorithms to get a triangulated surface. We typically have to remove redundant points and rearrange points so that the triangles have reasonable shape. Here are some pictures from an experiment done in December 2015 using a phone app. A Chimera statue which I photographed at the RISD in Providence was fed into a 3D mesh. (Unfortunately, that app is no more available. It was probably released for free to test the app, a typical path of software). If you look closely, you see that the software made it into a 2-dimensional manifold in the sense that every unit sphere is a circular graph with 4 or more elements. In other words, every unit sphere is a 1-dimensional discrete sphere. There are especially no tetrahedral structures. If you would count the number V of vertices, the number E of edges and the number F of faces, then V-E+F=2, because the model of the Chimera is actually a topological 2-sphere. Here is a more simplified model and here is a printout from a 3D printer. If we look what a scanner produces raw (I played myself once with a MS Connect scanner, like here) then the point clouds are at first rather messy. How can we still capture dimension from a point cloud which has many high dimensional simplices? In the winter 2020/2021, I exerimented with graph complements of circular graphs. These graphs are extremely interesting. I noticed first that many of them like have the cohomology of spheres. Indeed, all
are actually deformable to a d-sphere. But for these graphs, the unit spheres are now either contractible or co-dimension 1 spheres. In the graph complement of
which is a discrete Moebius strip, all unit spheres are contractible. In the graph complement of
all unit spheres are house graphs meaning that
is actually a 2-sphere in disguise. Indeed, it is a soft 2-sphere in the sense we have defined it. In the following video, I talk about this structure. The definitions are now fixed. They work. We are now in the process of proving theorems like that the Shannon product of two soft manifolds is a soft manifold. This is extremely important as we have now a compatibility with graph arithmetic. Also connection graphs of manifolds are soft manifolds. Connection graphs have nice properties (see for example this paper) or then compatibility with differential geometry: the curvature works well together with the Shannon product. Since the Shannon product has all the compatibilty we can dream of (spectral compatibility, cohomological compatibility, curvature compatibility) and produces a nice arithmetic generalizing the arithmetic of numbers, we really like this to work also for manifolds. But the Shanno product of two manifolds is not a manifold. The Shannon product of two circular graphs is a discrete torus in which every unit sphere is the connection graph of
.









