Stability of the Vacuum

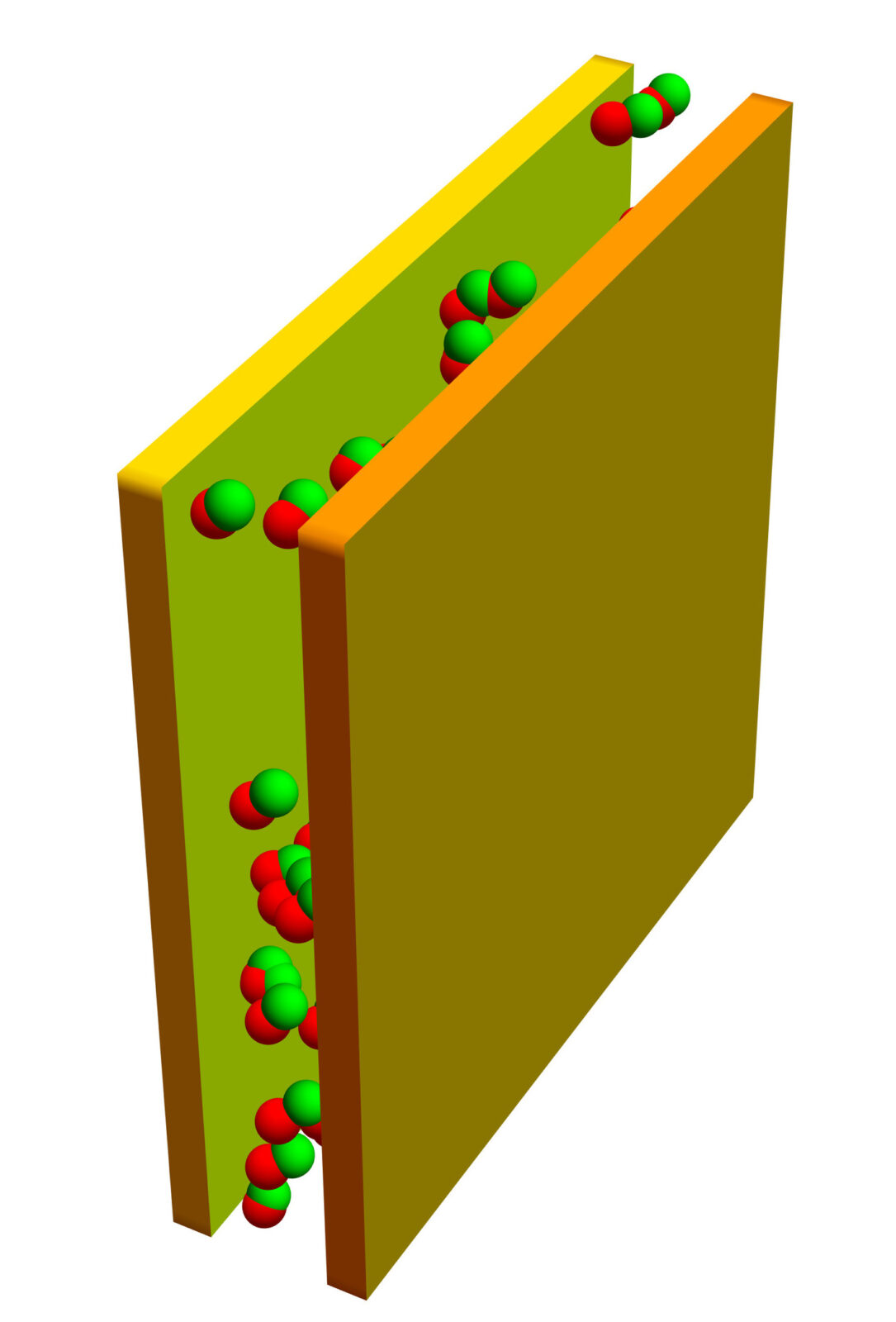
Explanations of the Casimir effect using common physics intuition like “polarization” (it originally was studied in the context of van der Waals forces) or “pressure” do not work. The reason in the case of the Casimir effect is that in the case of two planes or two cylinders the Casimir force is attractive while in the case of spherical shells the force is repulsive. The mathematical calculations involve divergent series which must be Zeta regularized. But when doing analytic continuations, one loses intuition. One has to use some ingenuity to argue that . That adding up all positive integers gives a negative value is something far from intuitive. As one can see in slides like this, the zeta function appears. One has to look at the difference of zero point energies with discrete energy values and continuum leading to the difference , which is of the form , when regularized gives . About intuition: how can one see intuitively that and and .

Side remark: I myself learned about divergent series in college and exactly remember the moment: I had been working as an undergraduate course assistant. Besides holding problem sessions and grading, we CAs had also been in charge to write homework problems and solutions for calculus courses. But it was quite easy: there was a “index card library” of problems, must have been thousands. There were organized like old library index cards. You could chose a topic and get a problem. One one side was the problem, on the other side was the solution. New problems were written mostly by graduate students or instructors. To make a problem set for the course, you would just put a couple of these index cards on a paper, and photocopy it. For the solution, one just would turn everything upside down and photocopy again. This could be done very fast. But already when I was a graduate student this started to get LaTeXed (I remember that Lutz Wilhelmy, who was a graduate student of Struve and was in the same office then mine had written a special LaTeX flavor to do that). Anyway, I had been in that course assistant room, when an other course assistant (who was writing a senior thesis in physics) came in and asked around, whether anybody knows about “Borel summation”. I did not. He explained that it is a method to sum divergent series and that it appears in the “Casimir effect”, to compute the force between two conducting (but uncharged) plates, a force which comes from the spontaneous generation of particles between. There are experiments from 1958, 1978 and 1997 with increasing accuracy. By the way, Wolfgang Pauli, who was well known for strong opinions (“not even wrong!”) had the following initial reaction to the Casimir effect: “absolute nonsense!”

I had been asked during dinner on Wednesday about the zero point energy. As a mathematician we can ignore the philosophical and even conceptional difficulties of a problem and look at simpler related problems. The zero point energy can be defined the infimum of the spectrum of some operator. It is also called the “ground state energy” but this is a bit misleading since if we look at operators without eigenvalues, there is no “state” = “unit vector” in the associated Hilbert space. For the free discrete Laplacian operator Lu(n) = u(n+1)+u(n-1)-2u(n) on for example, the spectrum is [0,4] with explicit density of states given by the probability density function of the arcsin distribution on [0,4]. It is the equilibrium measure of the Julia set of the quadratic map f(x)=x(4-x) which is conjugated explicitly to the tent map and so Bernoulli (as the induced dynamics on a Julia set always is). The bounded operator is conjugated by Fourier theory to the multiplication operator the inverse of which is the integrated density of states (CDF of the arcsin distribution in stats) which when differentiated gives the density of states . As a senior in college I already got interested in the topic, especially about the almost Mathieu operator latex 2\pi$. I would spend the next 13 years with trying to prove that the operator where is the orbit sequence of the Standard map (=equilibrium state of the Frenkel-Kontorova wave model) has determinant larger than 1 if c is large enough. The operator L as a random operator over a dynamical system is an element of a non-commutative type von-Neumann algebra and so has a natural determinant. By the Thouless formula and Pesin formula, a rephrases that the Kolmogorov-Sinai entropy of the Standard map is positive for large enough c. (The trace in tr(log(A)) for an operator is an average which makes sense as a Birkhoff average because are random variables. One calls such operators random operators even if there is nothing random associated with it; it is just a non-commuative random variable. Also in probability theory, a random variable is not a priori random at all. It is just a measurable function on a probability space.)

The Frenkel-Kontorova model is at the heart of many things I got exposed to in college. Juergen Moser gave a special one semester course on “Selected chapters in the calculus of variations” (to which I wrote the lecture notes in 1987 and retyped it around 2000 in LaTeX) in which he looked especially at Aubry-Mather theory, a beautiful (relatively trivial) modification of the KAM approach of finding smooth solutions of the nonlinear problem (*) for small . Linearization at gives in the Fourier picture the multiplication with which famously when inverted produces small divisors. If is Diophantine, meaning for all integers , one can invert the linearization operator on a subspace of smooth functions. But the inverted function is less smooth. If one uses a naive Newton iteration to find roots of the nonlinear problem (*) one loses smoothness in each step. Moser (similarly than Nash) found a way to modify the Newton step, adding some smoothing and show that one still can have convergence of that now called Nash-Moser iteration. In Aubry-Mather theory, one drops the requirement that q is smooth near q(x)=x but is monotone. The solutions produce Aubry-Mather sets. They exist for all and are minima of a variational principle but in general are Cantor sets. Often, the corresponding linearization operator is hyperbolic. I looked in my thesis also at the more general variational problem, where we take instead of an irrational rotation any ergodic dynamical system. Using the Aubry anti-integrable limit one can easily show (using the standard soft implicit function theorem) that for large c, a given dynamical system of finite entropy can be found within the standard map meaning that there are invariant measures of the Standard map for which the corresponding metric dynamical system is isomorphic to the given system. I called this “embedding of a metric dynamical system” in a topological dynamical system. Here is a paper of mine from August 1996. It shows also that there are arbitrary large c for which there are periodic points that are parabolic and so points which are elliptic (A result of Douarte which appeared around the same time). Typically near elliptic points, the twist theorem in KAM theory gives stable invariant islands. This also shows that it can not be true that for c large enough the Standard map is ergodic. It is not impossible however (even so unlikely) that there are values of c for which the Standard map is ergodic. I saw at UCLA (during a conference called “Dynamical systems Quo Vadis”) a talk of Sinai in which he claimed to have a proof ergodicity for some values of c. I also saw at Caltech (in a special seminar), Michael Herman give a proof that for some large values of c, the Standard map has positive entropy. I myself gave once a proof (maybe encouraged by such claims). All these claims have fallen. There is no value c known for which the Standard map has positive entropy. There is no value c known for which the standard map is ergodic. I’m still interested in the problem but do not work on it any more. It might be futile. What if the claim is false and the determinant is zero for all c? I also used it in teaching, like in this Unit 24: Chaos, of a course Math 22b given in Spring 2019 at Harvard. I have in 1999 written an online javascript calculator for the entropy of the Standard map. There is also a C program which produces (arbitrary large resolution) pictures of the standard map.

In the talk, I look mention again connection calculus. I talked about this several times already.

• Energy of a simplicial complex (published paper)
• Energized simplicial complex. (here on the blog) and ArXiv.
• Division algebra valued energized simplicial complexes ArXiv

Maybe something about the intro and exit footage done with my drone on Wednesay around 2 PM while making the 35 minute bike ride from our home in Arlington to Bentley (as a comparision, I need 20 minutes from home to Harvard) . The first introduction video shot was done in Lexington and in Waltham. When we lived on the other side of Arlington in School street, I would run frequently though the Rock Meadows, and Beaver Brook reservation, crossing so over from Arlington to Belmont via Waltham to Lexington. 20 years ago, the Avalon at Lexington Hills was still the abandoned Metropolitan State Hospital hospital (MET). It had been fascinating to run through that almost unreal place. The hospital buildings (arranged in a rectangular geometric form) were still there, chairs and equipment inside, but completely abandoned. Somebody made once a movie there, there were also artists who have used it as inspiration. There is also cemetery in the Beaver Brook reservation (with unnamed graves) which served the hospital. Apropos: John Nash had obtained treatment in the McLean Hospital which is close to the Rock Meadows (which is part of Belmont). There is still an abandoned and boarded Administrative building (still decorated with Graffiti like “climbing high?” near the tower) which needs renovation. The apartment buildings were built starting 2007. It had taken years and I also ran there through during construction. There is some youtube footage from the administrative building when it was not yet completely boarded up and which must have been used as a party place for kids. Pictures from the old place are hard to find. But they exist. But I found one picture and a panorama (displayed below). It shows the Cafeteria of the hospital. It must have been an amazing playground for a generation of kids in the neighborhood.One can read online about murder cases, about tunnels, about places where one could still see documents from patients. It looked a bit like Chernobyl.

And here is an areal picture, also from www.uer.ca, which shows the CTG building around 2000-2005, a time, when I had been running around there too. I would for example take a tour around and peak in.

The rest of the footage is done at Bentley university, a business school that was founded in 1917 and which moved in the sixties from Boston to Waltham. The big building with the tower is the Library. The talk had been in Lindsay Hall, in which the Wolfram Summer school resides over the summer. There had been dinner at the Student Center. Related to mathematics, the mathematician and historian Amir Aczel (1950-2015) was probably the most prominent mathematician who worked at Bentley college. I have seen some of his books like “Descartes Secret Notebook” which tells the fascinating story about the first appearance of the Euler formula V-E+F=2 in a lost notebook of Fermat who wrote in an encrypted form.

About the talk. There is a small typo on the right hand side of the board. where it is mentioned that the Fourier transform is , there is a missing. I should have mentioned that the anti-integrable limit easily shows that for larger c there are many solutions of as it is just a case for the standard (not-hard) implicit function theorem for nonlinear smooth maps in Banach spaces. The problem can be written as with . Now produces many measurable solutions like taking values each on sets of positive measure. These solutions continue to exist for by the standard implicit function theorem because for the linearization is invertible. This is Aubry’s argument. It is described also here.