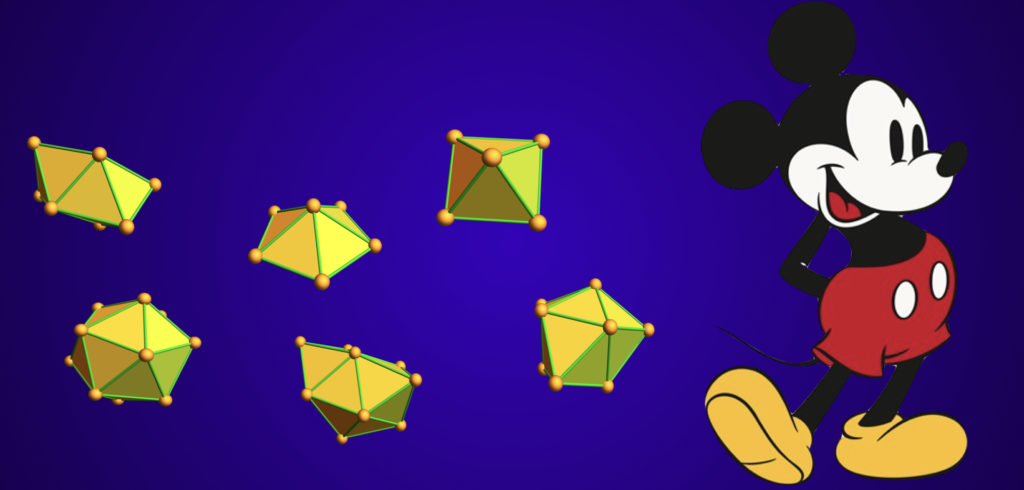
The Mickey Mouse theorem in dimensions 2 tells that positive curvature 2-graphs are 2-spheres.
Simple Sphere Theorem: For positive dimension d, a connected positive curvature d-graph is a d-sphere.
See preprint is on the ArXiv: “A simple sphere theorem for graphs. “
We are interested in discrete analogues of classical sphere theorems in differential geometry where purely combinatorial notions are used and Euclidean space never enters. Actually, for us, the continuum, or infinity does not even have to exist.
To make this theorem easy to prove we define d-graphs and d-spheres recursively. These definitions discrete manifolds and discrete spheres which origins from the 1990ies, in frame works called digital topology. It completely ignores the difficulties and confusions one has to work with when dealing with triangulations of manifold. The idea is that one recursively (with respect to dimension) defines what a manifold or sphere is and uses the language of graphs which is intuitive. Anybody who can read a Mickey mouse comic can also understand what a graph is: it is a collection of nodes connected by edges.
Now, the definition going back to Evako (Ivashchenko) is recursive: Start with the empty graph 0 (zero) and call it the (-1) sphere. Also use the 1 point graph 1 (one) and declare it to be contractible. Now, contractibility, d-graph and d-sphere are defined recursively: a graph is a d-graph if every unit sphere is a (d-1) sphere and a d-sphere is a d-graph for which after removing a vertex x, we end up with a contractible graph G-x. A graph is contractible if there exists a vertex x such that both S(x) and G-x are contractible.
A graph is said to have positive curvature if every embedded wheel graph has positive curvature. This is a very strong condition. Indeed, it will imply that all positive curvature graphs have diameter less or equal than 3. It turns out that for d larger than 1, a positive curvature graph has diameter less or equal than 3. This will imply that the graph is a union of two balls and so, as in the continuum has to be a sphere. The positive curvature condition is so strong that we can not realize a projective plane as a positive curvature graph.
This Mickey Mouse theorem is already for a while in the making. In 2011, a list of positive curvature 2-graphs has been included in this preprint on discrete Gauss-Bonnet-Chern . I had hoped to prove such a theorem with a relaxed curvature condition assuming some additional pinching but that is harder. What the heck. We can also resist the temptation and keep things simple. I call the theorem the simple sphere theorem or Mickey mouse theorem.
The proof of the theorem is simple. The positive curvature assures that the diameter of the graph is either 2 or 3 (it is here where all the recursive definitions play a role). We prove a geomag lemma which allows to extend a two dimensional surface with boundary. This extension of a surface does not assume that the extension is an embedding, it can be an immersion but it will be one of the 6 positive curvature 2-graphs. The small diameter implies that the graph can be covered with 2 balls. The ball of radius 2 in a graph of positive curvature is still a ball (this uses the positive curvature condition). Having covered a graph with two balls makes it a sphere. This is completely analogue to the continuum: if a compact manifold is covered by two balls, then it is a sphere. In the discrete it is much easier: just use the definition of contractibility to show that puncturing G produces a contractible graph: reduce one of the balls until the second ball is there and then use the definition of contractibility of the second ball. This establishes that G is a sphere.

Donald, Daisy, Minnie, Mickey, Pluto and Goofy

Donald, Daisy, Minnie, Mickey, Pluto and Goofy admiring a positive curvature graph