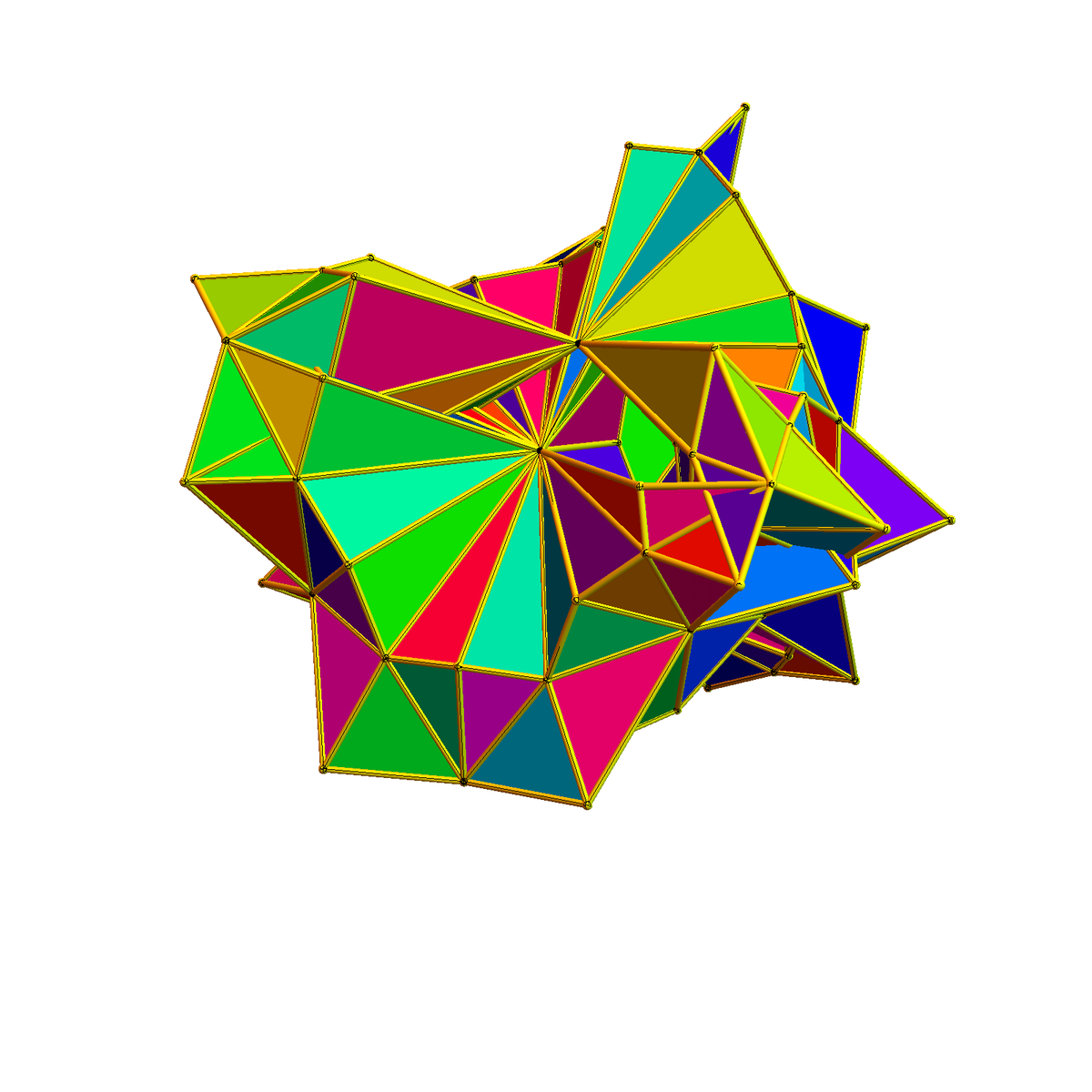
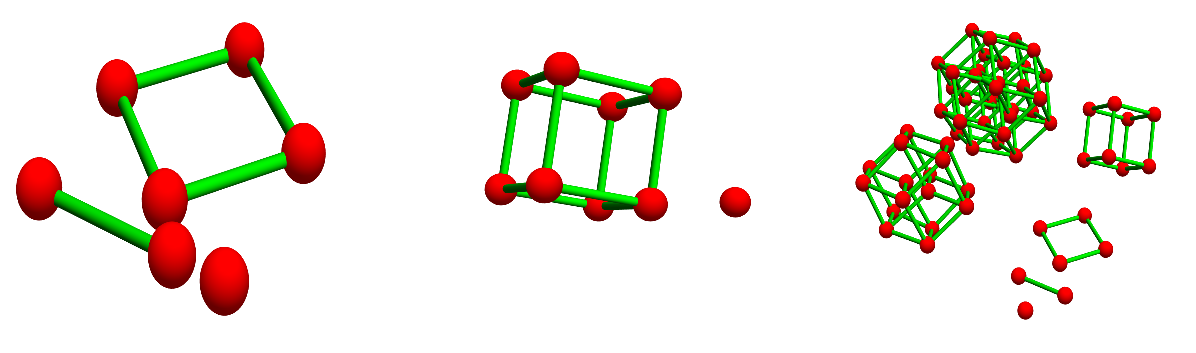
Kruskal-Katona
If is a finite abstract simplicial complex, a finite set of non-empty sets closed under the operation of taking non-empty subsets, we can ask about what f-vectors can occur if counts the number of sets of cardinality k in G. The case of the complete complex with gives a hint …